F(x) = x – 2 Consider c > 1 and f(c) = c – 2 \(\lim_{x \rightarrow c}f(x) = \lim_{x \rightarrow c}x2 = c2\) Thus, the function f(x) is continuous at all real numbers greater than 1 Case 3 When x = 1, f(x) = x 2 Consider c = 1, now we have to find the lefthand and righthand limits LHL \(\lim_{x \rightarrow 1^}f(x) = \lim_{x \rightarrow 1^}x2 = 12=3\) RHLFunctions are given letter names The names are of the form f(x) which is read "f of x" The letter inside the parentheses, usually x, stands for the domain set The entire symbol, usually f(x), stands for the range set The orderedpair numbers become (x, f(x))So we can see that when x is equal to zero, f of x is equal to one, so g of x should be equal to two because it's two times f of x So g of x is going to be equal to Or g of zero, I should say, is going to be equal to two What about when at x equals, we'll say when x equals three When x equals three, f of x is negative two
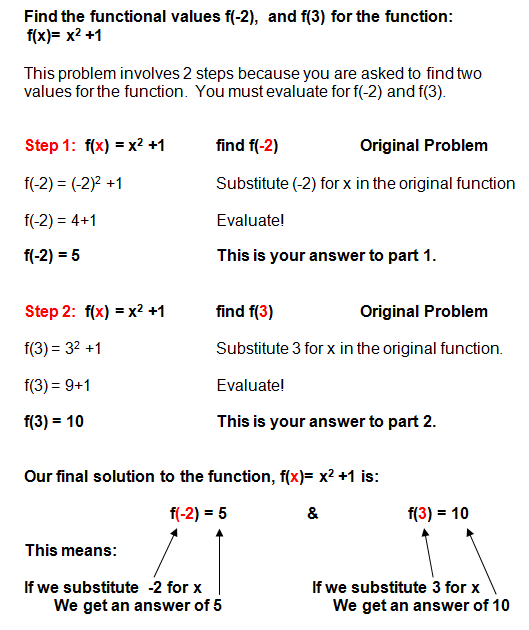
Evaluating Functions
